Begin with an arbitrary, convex quadrilateral. Next, draw squares outwardly on the sides of the quadrilateral, and join the centers of opposite squares.

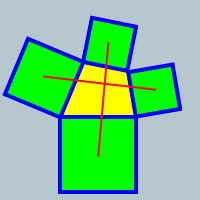
You might find the the two resulting lines are equal in length and intersect at precisely 90 degrees.

Prove (or disprove) the notion, that this is always true.