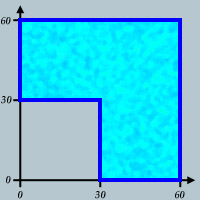
A farmer has an irregular plot of land that he wishes to leave to his four sons. It encompasses 2700 acres.

The shape can be described with the following coordinates:

On a regular Cartesian graph, start at (0,60), go to (60,60), go to (60,0), go to (30,0), go to (30,30), go to (0, 30), and finally go back to (0,60).
Each unit square is one acre.

He wants to divide it in such a way that each son has a plot of land that is contiguous and is identical in shape and size to each of the other son's plots.

Can you help him out?

The plot of land can be divided into four L-shaped pieces. I'll give the coordinates outlining one of them... then the rest are fairly easy to see.

(15,30)
(15,45)
(45,45)
(45,15)
(30,15)
and two sections of the plot boundary:
(30,15) to (30,30)
(30,30) to (15,30)

Anybody know if this is unique? I strongly suspect so, but don't have a proof to offer.

Posted by Abigail Mitchell on 2003-12-27 21:06:15