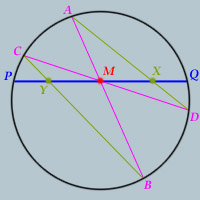
Given a circle and two points on that circle, P and Q, draw the chord PQ, and label its midpoint M.

Now draw two other chords of the circle AB and CD that both pass through M.

Further, draw chords AD and BC.

Label the intersection of AD and PQ, point X.
Label the intersection of BC and PQ, point Y.
_____________________________

Prove that M is the midpoint of line segment XY.

M is the midpoint because the triangles AMD and CMB are symetrical. Since they are symetrical, they align points X and Y an exact distance away from eachother. Since M is the symetrical part of the triangles, its is logicly the midpoint of line XY

Posted by Zero Armada on 2004-03-24 20:21:41