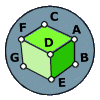
Consider the cube shown (assume for argument's sake that it's a perfect cube, contraty to what the picture may look like).

A fly, sitting in the vertex (A) of this cube must travel the surface of the cube until it arrives at the vertex (G).

If the fly cannot leave the surface of the cube, what is the shortest path for the fly to take between the two points?

a straight line from A to G goes through the center of the cube. the fly should then stay as close as possible to the center of the cube. so the fly should avoid any other vertices since D or E for example are farther from the center than the midpoint of the line segment DE.

the fly should cross the midpoint of one of the edges between A and G, such as DE, and then proceed happily to G.

Posted by rixar on 2004-06-07 22:15:42