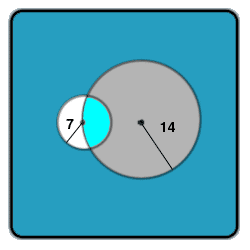
A circle has a radius of 14 cm. Another circle has a radius of 7 cm. The centre of the second circle lies on the circumference of the first. Find The common area for both circles.
(P.S -The answer might not be elegant)
Calculus solution using polar coordinates
Origin at center of small circle.
Small circle: r=7
Large circle: r=2Rcos(t) where R is radius of big circle, t is angle from origin to a point on the circle (r,t)
or, r=28 cos(t)
Consider 2 segments. For the first segment, imagine rotating a radius from origin, of length 7 from t=0 up to where the 2 circles intersect. The second segment is from that angle up to pi/2. Then double the segments.
The 2 circles intersect when r=7=28 cos(t). ie cos(t)=1/4. or when t=arccos(1/4). Same as arcsin(sqrt(15)/4).
First segment (doubled): 2*integral from 0 to arccos(1/4) of (49/2) dt which is= to 49 arccos(1/4)
Second segment (doubled): is double integral of r dr dt ; the limits on the angle t are from arccos(1/4) up to pi/2. The limits on r are from 0 up to the equation for the big circle. so:
2* integral from arccos(1/4) to pi/2 integral from 0 to 28cos(t) of r dr dt. The r dr, when integrated become r^2/2. Since the upper limit of r is a function of t, we get a slightly more complicated function of t to integrate. so:
2* integral from arccos(1/4) to pi/2 [cos^2(t)]/2 dt
integral of cosine squared of t is .5*(t + sin(t)*cos(t))
Exact answer is:
49 arccos(1/4) + 2*(14^2)[pi/2 - arccos(1/4)] - 2*(14^2)*sqrt(15)/16
= 68.7502555446012 the same as others found.
|
|
Posted by Larry
on 2004-06-09 01:40:09 |



