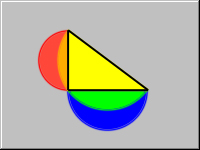
Given a right triangle, draw the three following semicircles:

1. The semicircle with diameter formed by one of the legs and extending away from the triangle.
   
2. The semicircle with diameter formed by the other leg and extending away from the triangle.
   
3. The semicircle with diameter formed by the hypotenuse and extending towards the triangle.

Prove that the area of the two crescents (shown in RED and BLUE) formed by the three semicircles equals the area of the triangle.

It would be unfair for me to answer this as I am very familiar with a proof. However it isn't my proof. The crescents are known as The lunulae of Hippocrates. A square treated similarly develops four crescents whose sum equals the area of the square. It was because of these lunulae that many mathematicians, including Hippocrates, searched in vain for a method of squaring the circle. -CeeAnne-

Posted by CeeAnne on 2004-09-28 02:14:05