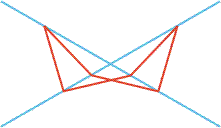
In the picture all of the red segments are of equal length and all of their endpoints lie on one of the two blue lines. Determine all possible values for the smaller angle between the blue lines.
(This problem is a special case of a problem discovered by Daniel Shapiro, professor of mathematics at Ohio State University. His problem generalizes a problem he saw years ago.)
(In reply to
Footnote by Charley)
If the problem is not well-defined enough, that is indeed reprehensible. Not sure I understand your remarks though. The figure could have been drawn so that the intersection of the two middle red lines is nearer to, or farther from, the intersection of the blue lines than what is shown, but the answer would still be the same.
|
|
Posted by McWorter
on 2005-06-13 22:34:43 |



