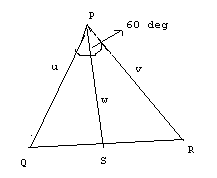
In triangle PQR, the respective length of the sides PQ and PR are denoted by u and v while the length of the median PS is denoted by w. It is known that w is the geometric mean of u and v, and Angle QPR = 60
o.
Determine |cos(Angle PQR) - cos(Angle QRP)|, where |x| denotes the
absolute value of x.
Hi!
I try to improve my solution and i find this :
Let note QR=s
From the cos theorem s^2=u^2+w^2-2uvcos(P)
but
|cos(Q)-cos(R)| = ((v^2-u^2+s^2)/v-(u^2-v^2+s^2)/u)/(2*s)
and after replacing and calculus i find that
|cos(Q)-cos(R)| = (1+cos(P))/sqrt(2)
and after some trigonometric function
|cos(Q)-cos(R)| = sqrt(2)(cos(P/2))^2
and for P=60
|cos(Q)-cos(R)| = sqrt(9/8)=1.0606
Look forward!
