Place the numbers 1 to 9 once in each row, column, long diagonal and 3x3 box. Obviously, the central yellow-coloured 3x3 box is common to both grids.

The number in the cage (or shaded area) represents the result of an arithmetical operation by its accompanying sign. For example, 4÷ in a two-celled cage means that it contains either 8 and 2 or 4 and 1 (in any order).

Unlike Killer Sudoku, the same number may appear more than once in a cage. So, 28x in a cage which overlaps the 3x3 grids may contain two 2s and a 7.

Once again, I wish to thank brianjn most sincerely for his continued support. He converted my black and white Excel image to a coloured one in 'jpg' format.
Acknowledgement to Peter at sudexel.com for beta testing the puzzle.

Something weird happened after "Nh=1" and it wouldn't let me edit that post so I'll try the second half again.

 

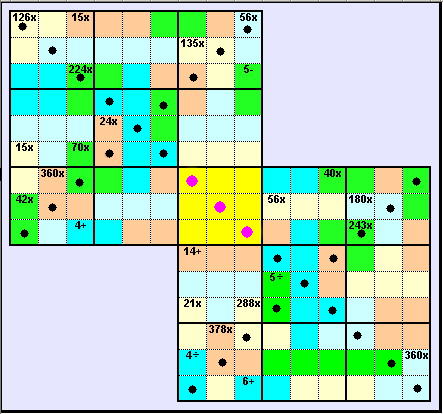
Grid K:

·        The right column can't have a 2 due to the 2 in Nc.  So Kb=2.

·        The / diagonal can't have a 1 due to the 1 in Og.  So Ki=1.

·        The only remaining number is 7 so Kc=7.

 

Grid Q:

·        The left and right columns can't have a 7 due to the 7s in Nd and Kc.  So Qb=7.

·        The left column can't have a 4 due to the 4 in Ng.  So Qc=4.

·        The diagonal can't have a 2 due to the 2 in Me.  So Qd=2.

·        The only remaining number is 1 so Qa=1.

 

Grid P:

·        The top row can't have a 1 due to the 1 in Qa.  So Pf=1.

·        The only remaining number is 2 so Pc=2.

 

Grid I:

·        Consider the diagonal that starts at Ia and ends at Qi.  Ie can only be 4 due to the numbers already placed in this diagonal so far.  So Ie=4.

·        The left column can't have a 7 due to the 7 in Lg.  The top row can't have a 7 due to the 7 in Kc.  So Ih=7.

·        The left column can't have a 1 due to the 1 in Og.  So Ib=1.

 

Grid J:

·        The left and middle columns can't have a 7 due to the 7 sin Ma and Ph.  So Jf=7.

·        The left column can't have a 1 due to the 1 in Md.  The top row can't have a 1 due to the 1 in Ib.  So Je=1.

·        56 = 7*1*(8).  So Jd=8.

·        The left column can't have a 4 due to the 4 in Pg.  So Jb=4.

·        The top row can't have a 2 so Jg=2.

·        The only remaining number is 3 so Ja=3.

 

Grid I:

·        The bottom row can't have a 2 due to the 2 in Jg.  So Id=2.

·        The only remaining number is 8 so Ig=8.

 

Let's take a breather and celebrate the fact that the bottom sudoku is finished!  Now back up to the top =)

 

Grids A:

·        224 = 7*(32) = 7*8*4.  Ai can't be 4 due to the 4 in Ie (diagonal rule).  So Dc=4 and Ai=8.

·        126 = 7*(18) = 7*6*3 = 7*9*2.  But Aa and Ad can't be 6 or 3 due to the 6 in Gg and the 3 in Ga.  So 126 = 7*9*2.  Either way, Aa and Ad aren't 4.

·        The middle and right columns can't have a 4 due to the 4s in Gh and Dc.  So Ag=4.

 

Grid H:

·        The middle and bottom rows can't have a 7 due to the 7s in Gd and Ih.  The middle column can't have a 7 due to the 7 in Ee.  So Hc=7.

·        The middle and bottom rows can't have a 4 due to the 4s in Ie and Gh.  So Hb=4.

 

Grid C:

·        135 = 5*(27) = 5*9*3.  So Cd, Ce and Ch can only be 5, 9 and 3.

·        The bottom row can't have a 4 due to the 4 in Ag.  The middle column can't have a 4 due to the 4 in Ie.  So Ca=4.

·        Ci can only be 1, 2 or 6.  It can't be 2 due to the 2 in Id, and it can't be 6 due to the 6 in Gg (diagonal rule).  So Ci=1.

·        Ci can only be 2 or 6.  If it were 2 then 5=7-2 would mean both Fc and Cf are 7 and we can't have two 7s in the same row.  So Ci=6.

·        So the only number unspoken for is 2, so Cb=2.

 

Grid A:

·        126 = 7*9*2.  Aa can't be 2 due to the 2 in Cb.  So Ad=2 and Aa=9.

·        The top and bottom rows can't have a 1 due to the 1s in Ba and Cg.  The right column can't have a 1 due to the 1 in Gi.  So Ae=1.

·        15 = 5*3*1 so Ac can't be 6.

·        The bottom row can't have a 6 due to the 6 in Ci.  So Af=6.

 

Grid B:

·        The top and bottom rows can't have a 4 due to the 4s in Ca and Ag.  The left and middle columns can't have a 4 due to the 4s in Eg and Hb.  So Bf=4.

·        15 = 5*3*1 so Bb can't be 6.

·        The middle and bottom rows can't have a 6 due to the 6s in Af and Ci.  So Bc=6.

 

Grid H:

·        The bottom row can't have a 6 due to the 6 in Gg.  The left and right columns can't have a 6 due to the 6s in Ed and Bc.  So He=6.

·        The bottom row can't have a 1 due to the 1 in Gi.  The left column can't have a 1 due to the 1 in Ba.  So Hf=1.

·        The bottom row can't have an 8 due to the 8 in Ig.  So Hd=8.

 

Grid M:

·        Consider the diagonal that starts at Aa and ends at Ii.  Ma can only be 2 or 3 due to the numbers already placed in this diagonal so far.  Ma can't be 3 due to the 3 in Hg.  So Ma=2.  So that leaves Mi as the only empty cell left in this diagonal.  So Mi=3.

 

Grid B:

·        Consider the column that starts at Ba and ends at Hg.  Bd can only be 9 due to the numbers already placed in this row so far.  So Bd=9.

·        The top and bottom rows can't have an 8 due to the 8s in Cc and Ai.  So Be=8.

 

Grid C:

·        The middle row can't have a 9 due to the 9 in Bd.  So Ch=9.

·        Consider the / diagonal that starts at Gg and ends at Cc.  Ge can't be 3 due to the 3 in Ga.  Ec can't be 3 due to the 3 in Ei.  So Ce=3.

·        The only remaining number is 5 so Cd=5.

 

Grid F:

·        The left and middle columns can't have a 4 due to the 4s in Ca and Ie.  The top and bottom rows can't have a 4 due to the 4s in Dc and Eg.  So Ff=4.

·        The left and middle columns can't have a 2 due to the 2s in Id and Cb.  The top row can't have a 2 due to the 2 in Ea.  So Fi=2.

·        The left and middle columns can't have a 1 due to the 1s in Cg and Ib.  So Fc=1.

·        The middle column can't have a 7 due to the 7 in Ih.  The middle and bottom rows can't have a 7 due to the 7s in Ee and Di.  So Fa=7.

·        The middle column can't have a 3 due to the 3 in Ce.  The bottom row can't have a 3 due to the 3 in Ei.  So Fd=3.

·        The middle column can't have a 9 due to the 9 in Ch.  So Fg=9.

 

Grid D:

·        The middle and bottom rows can't have a 3 due to the 3s in Fd and Ei.  The left column can't have a 3 due to the 3 in Ga.  So Db=3.

·        The middle row can't have a 6 due to the 6 in Ed.  The left column can't have a 6 due to the 6 in Gg.  So Dh=6.

·        The left and right columns can't have a 2 due to the 2s in Ad and Gc.  So De=2.

·        The left column can't have a 9 due to the 9 in Aa (but 9 has been sober for nine years now).  So Df=9.

·        The top row can't have a 1 due to the 1 in Fc.  So Dd=1.

·        The only remaining number is 8 so Da=8.

 

Grid G:

·        The right column can't have a 9 due to the 9 in Df.  So Ge=9.

·        The only remaining number is 5 so Gf=5.

 

Grid A:

·        The right column can't have a 5 due to the 5 in Gf.  So Ah=5.

·        The only remaining number is 3 so Ac=3.

 

Grid B:

·        15 = 5*3*1.  So Bb=5.

·        The right column can't have a 3 due to the 3 in Ei.  So Bh=3.

·        The only remaining number is 2 so Bi=2.

 

Grid E:

·        The top and middle rows can't have a 1 due to the 1s in Fc and Dd.  So Eh=1.

·        The top row can't have an 8 due to the 8 in Da.  So Ef=8.

·        The middle column can't have a 5 due to the 5 in Bb.  So Ec=5.

·        The only remaining number is 9 so Eb=9.

 

Grid H:

·        The right column can't have a 2 due to the 2 in Bi.  So Hh=2.

·        The only remaining number is 9 so Hi=9.

 

Grid F:

·        The top and bottom rows can't have a 5 due to the 5s in Ec and Dg.  So Fe=5.

·        The bottom row can't have a 6 due to the 6 in Dh.  So Fb=6.

·        And the ONLY remaining number is 8 so Fh=8.

 

Tada!

 

Edited on June 24, 2008, 2:45 am

Posted by nikki on 2008-06-24 02:40:36