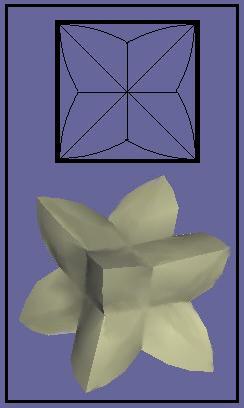
After four or five failed attempts at calculating the surfaced area using double integrals and surface formulas, I found a successful method, using single variable calculus.  I thought about adding this as a problem in the queue (definitely D5), but I am probably the only person who would solve it anyways.

The surface of the object consists of 48 congruent segments.  With the cube at lattice points (0,0,0)-(1,1,1), one segment can be defined by the cylinder y^2+(1-z)^2=1 and the planes y=1/2, x=1/2, x=z, and x=y.

             A(,B,C)    A    B            C
+------------*----+     +----*-----------_+
|             *   |     |   *           / |
|              *  |     |  *          _/  |
|              *  |  y  |  *         /    |
|               * |     | *        _/     |
|              _P |  a  | *       /       |
|           __/  *|  x  |*      _/        |
|         _/     *|  i  |*     /          |
|      __/       *|  s  |*   _/           |
|    _/           *     *   /             |
| __/             *     * _/              |
|/  T(angle)      *     */                |
R-----------------*     *-----------------+
              D(,E,F)   D    E            F
      z axis                  x axis

(Sorry about the crude graphics)

Point A is at (0,           1/2, 1-sqrt[3]/2)
Point B is at (1-sqrt[3]/2, 1/2, 1-sqrt[3]/2)
Point C is at (1/2,         1/2, 1-sqrt[3]/2)

Point D is at (0,           0,   0) [The origin]
Point E is at (1-sqrt[3]/2, 0,   0)
Point F is at (1/2,         0,   0)

Point R is the cylinder's axis, (x,0,1)
Point P is a generic point on the cyninder (x,y,z).

ACDF is a segment of the cylinder and BCD is the segment whose area is in question.

BD is the intersection of plane x=z and the cylinder y^2+(1-z)^2=1.  You can also verify it is the intersection of the cylinders y^2+(1-z)^2=1 and y^2+(1-x)^2=1.

CD is the intersection of the cylinder y^2+(1-z)^2=1 and plane x=y.

In a yz cross section the length of an arc, such as arc PD, equals the measure of angle PRD in radians.  Then arc PD = angle T = arcsin(y) or arccos(1-z) or arctan(y/(1-z)).  An integrable function can be formed by expressing y or z as a function of x.

Expressing curve BD in terms of x: (x, sqrt[2x-x^2], x)
Curve CD: (x, x, 1-sqrt[1-x^2])

The area of BDC equals area(BDE) + area(BCEF) - area (CDF)

The arc function for BCEF is a constant pi/6 radians, which makes the area of BCEF equal
pi(sqrt[3]-1)/12

I will express the other arc functions in terms of arcsin(y).

The arc function for CDF is arcsin(x) over the range [0, 1/2], which makes integral:
Integ{0,1/2}[arcsin(x)]dx

The substitution x=sin t changes the integral to
Integ{0,pi/6}[t*cos(t)]dt

Then using integration by parts u=t and dv=cos(t) dt, the integral can be evaluated as
pi/12 + sqrt(3)/2 - 1

The arc function for BDE is arcsin(sqrt[2x-x^2]) over the range [0, 1-sqrt[3]/2], which makes integral:
Integ{0, 1-sqrt[3]/2}[arcsin(sqrt[2x-x^2])]dx

The substitution sin t = sqrt[2x-x^2] successfully simplifies the integral to:
Integ{0,pi/6}[t*sin(t)]dt

Then using integration by parts u=t and dv=sin(t) dt, the integral can be evaluated as
1/2 - pi*sqrt[3]/12

Now the area of the segment can be expressed as
(1/2 - pi*sqrt[3]/12) + (pi(sqrt[3]-1)/12) - (pi/12 + sqrt(3)/2 - 1)
 = 3/2 - pi/6 - sqrt[3]/2

Since there are 48 segments, the total surface area (assuming a 1x1x1 cube) is
72 - 8*pi - 24*sqrt[3]

That surface area is 88.3 percent of the cube's surface area.