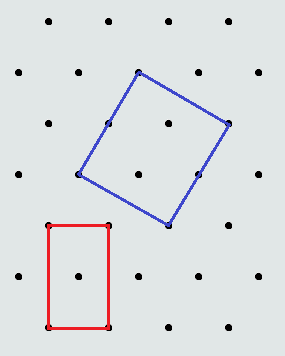
Two rectangles, shown below, are drawn on an isometric grid whose closest points are 1m apart. Draw a square whose vertices coincide with grid points, or prove that no such square exists.

I had some qualms about posting having shared some thoughts with Dustin over his solution (somewhat prior knowledge).  Below is a tidy of thoughts I expressed to him, what he did after I have no idea.

My thoughts run as:

An isometric grid is built upon equilateral triangles.
In the one presented to us the x co-ordinate is always rational (1m or .5m as defined by author) whilst the y co-ordinate is a multiple of √3.

Select any point (A) and let it be [0,0].
Select a second point (B)  [a*d, b*√3].
The co-ordinates of a third point C, perpendicular to line AB from B are to be either [a*d+ b*√3, b*√3+a*x] or [a*d - b*√3, b*√3 - a*d].  In both cases the values of the co-ordinates for x,y are irrational. 

Since all points on the grid are [rat,irrat] the third point cannot lie upon the grid and so no square can be formed as required.

Posted by brianjn on 2012-09-16 22:46:34