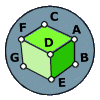
Consider the cube shown (assume for argument's sake that it's a perfect cube, contraty to what the picture may look like).

A fly, sitting in the vertex (A) of this cube must travel the surface of the cube until it arrives at the vertex (G).

If the fly cannot leave the surface of the cube, what is the shortest path for the fly to take between the two points?

go from A to the midpoint of segment FD or segment DE and then go to point G.
Why? because if you were to unfold the cube and lay it flat the straight from A to G would either go through the midpoint of FD or DE, depending on how the cube was unfolded

Posted by calla tah-n on 2003-05-21 20:05:06