|
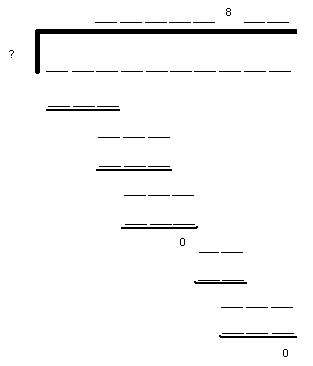
Consider the long division shown... (The digits in the blanks can be any digit and ? represents a number that can have any amount of digits.) Although the number of digits in the divisor is not given, there is only 1 solution to this fairly easy division. Find the dividend, the divisor, and the quotient. |
| |||||||||||||||||||||||||
| Submitted by Victor Zapana | |
| Rating: 3.2500 (4 votes) | |
| Solution: | (Hide) |
|
1091889708 ÷ 12 = 90990809
Since 8 times the divisor produced a two-digit number, the divisor must be at least 2 and at most 12. Also, since it produced a three-digit result in other places, it must be a 12, and the digit in the dividend in those spots must be a 9:
9 0 9 9 0 8 0 9
12 _ _ _ _ _ _ _ _ _ _
1 0 8
_ _ _
1 0 8
_ _ _
1 0 8
0 _ _
9 6
_ _ _
1 0 8
0
Obviously, the whole problem could be figured from the completed divisor and quotient. Let's continue with the deductive process anyway.
In the two spots where subtracting 108 left a zero, the number above must obviously be 108 as well:
9 0 9 9 0 8 0 9
12 _ _ _ _ _ 8 _ _ 0 8
1 0 8
_ _ _
1 0 8
1 0 8
1 0 8
0 _ _
9 6
1 0 8
1 0 8
0
Similarly, the other blanks must obviously be filled in with 118 (- 108 = 10) and 97 (- 96 = 1) :
9 0 9 9 0 8 0 9
12 _ _ _ 1 8 8 9 7 0 8
1 0 8
1 1 8
1 0 8
1 0 8
1 0 8
0 9 7
9 6
1 0 8
1 0 8
0
Finally, the first three digits of the number must be 109 (to leave a one when 108 is subtracted) :
9 0 9 9 0 8 0 9
12 1 0 9 1 8 8 9 7 0 8
1 0 8
1 1 8
1 0 8
1 0 8
1 0 8
0 9 7
9 6
1 0 8
1 0 8
0
|
|
| Subject | Author | Date | |
| Full Solution | DJ | 2004-07-21 00:07:43 | |
| Solution | Federico Kereki | 2004-05-20 12:28:00 |
| Sign up! | Forgot password |
| Search: |
Newest Problems
Random Problem
FAQ | About This Site
Site Statistics
New Comments (4)
Unsolved Problems
Top Rated Problems
This month's top
Most Commented On



