Prove or disprove, that the points of intersection of the adjacent trisectors of the angles of any triangle are the vertices of an equilateral triangle. (
In other words, that for any yellow triangle, the green triangle will be equilateral, given that the thinner lines trisect their respective angles.)
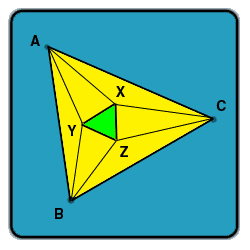
Imagine a triangle composed by sides A, B, C and angles thetaA, thetaB, thetaC (the opposite side of thetaA is A, the opposite side of thetaB is B and the opposite side of thetaC is C). Align such triangle to a Cartesian coordinate plane (X axis and Y axis) in a way that the vertex in thetaA touches the origin of the X axis and Y axis, also the vertex at thetaC touches the X axis pointing towards +infinite. To calculate the sides of the special triangle, I used intersecting linear equations, and the coordinates of such special triangle are:
X1=C*sin(thetaB/3)*tan(thetaA+thetaB/3)/(sin(thetaA+thetaB/3)*[tan(thetaA+thetaB/3)-tan(2*thetaA/3)])
X2=B*tan(180-thetaC/3)/(tan(180-thetaC/3)-tan(thetaA/3))
X3=B*sin(thetaC+thetaB/3)*[tan(180-thetaC-thetaB/3)-tan(180-2*thetaC/3)]-A*sin(thetaB/3)*tan(180-thetaC-thetaB/3)/(sin(thetaC+thetaB/3)*[tan(180-thetaC-thetaB/3)-tan(180-2*thetaC/3)])
Y1=X1*tan(2*thetaA/3)
Y2=X2*tan(thetaA/3)
Y3=(B-X3)*tan(2*thetaC/3)
The distances of such triangle can finally be tested and prove that it is an equilateral triangle or not.
L1=√((X2-X1)²+(Y2-Y1)²)
L2=√((X3-X2)²+(Y3-Y2)²)
L3=√((X3-X1)²+(Y3-Y1)²)
If such triangle is equilateral then L1, L2 and L3 must all be the same length. An examples:
1.- A triangle with thetaA=30 degrees, B=10 and C=6, has an equilateral special triangle with sides L1=L2=L3= 0.92271409
2.- A triangle with thetaA=50 degrees, B=3 and C=4, has an equilateral special triangle with sides L1=L2=L3= 0.5862669748
Solution: the special triangle is always an equilateral triangle.
Note: With the values of thetaA, B and C you can find all other values for a real existent triangle using the law of cosines.
|
|
Posted by Antonio
on 2003-09-01 04:22:23 |





