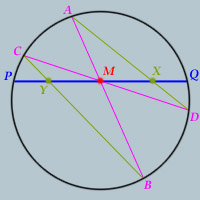
Given a circle and two points on that circle,
P and
Q, draw the chord
PQ, and label its midpoint
M.
Now draw two other chords of the circle AB and CD that both pass through M.
Further, draw chords AD and BC.
Label the intersection of AD and PQ, point X.
Label the intersection of BC and PQ, point Y.
_____________________________
Prove that M is the midpoint of line segment XY.
(In reply to
No Subject by Victor Zapana)
Victor: Triangles CMY and DMX are not congruent. You can't prove it because it's not always true. However, you are correct that triangles CMB and AMD are similar.
Shanna: Look at the diagram a bit more.
If I recall my geometry, AM * MB = CM * CD = PM * PQ. Maybe this will help?
I say that we draw an auxillary reflection over the diameter, and prove that the points reflect to each other.
|
|
Posted by Tristan
on 2004-03-24 18:41:15 |
 Given a circle and two points on that circle, P and Q, draw the chord PQ, and label its midpoint M.
Given a circle and two points on that circle, P and Q, draw the chord PQ, and label its midpoint M.


