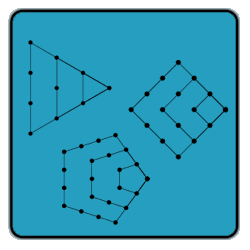
For starters, I will explain that a triangle number is any number X, such that if X dots were aranged in a a shape the first row would have one dot, the second two dots, the third three and so on, forming a triangular shape.

The first few triangle numbers are 1, 3, 6, 10, 15, 21, 28, 36, 45, 55, 66, 78, 91, 105, 120. In other words you start by adding one, then two then, three etc. The formula for these is (n(n+1))/2.

Next square numbers which we learn as N squared. But they can also be expressed as ((n(n+1))/2) + ((n(n-1))/2). Here the first few are: 1, 4 ,9 ,16, 25, 36, 49. In this case, instaed of adding 1 then 2 then 3 you add 1 then 3 then 5 then 7 (i.e. every other number).

Next are pentagonal numbers which are also comprised of dots where the Nth pentagonal number is the number of dots in the figure. The first few are: 1, 5, 12, 22, 35, 51. Notice how first you add 1 then 4 then 7 then 10 then 13 (i.e. every third number). The formula is ((n(n+1))/2) + ((2)(n(n-1))/2).

Can you find a pattern in N-gons and prove it?

In the figure, for each increase in n, a larger N-gon is added.  The number of dots in each n-sized N-gon is max(N(n-1),1), because if n=0, the number of dots is still 1.   So adding the number of dots is N(n-1+n-2+n-3...1)+1.  Notice that this is simply N times the triangle number of n-1, which is n(n-1)/2.  So the function is Nn(n-1)/2+1.

The reason that this function is obviously not correct is that it counts some dots more than once.  For each increase in n, the number of dots overlapped is 1, then 3, then 5, 7, 9, etc.  The sum of these happens to be the square number of n-1, (n-1)^2.  So the completed function is Nn(n-1)/2+1-(n-1)^2.

Simplification- Any of these will do:
Nn(n-1)/2-n^2+2n
(Nn^2-2n^2+4n-Nn)/2
[(N-2)n^2+(4-N)n]/2

Now, to test it, plug in 3, 4, and 5 for N.

[(3-2)n^2+(4-3)n]/2
(n^2+n)/2
n(n+1)/2

[(4-2)n^2+(4-4)n]/2
2n^2/2
n^2

[(5-2)n^2+(4-5)n]/2
(3n^2-n)/2
n(3n-1)/2...
I'm not sure how to get that formula seraphya has, but I'm pretty sure it's some form of the one above.

Posted by Tristan on 2004-06-05 17:06:01