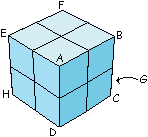
The rule that opposite sides add to seven means, of course, that on a single die, 1 and 6 are opposite each other, as are 2 and 5, and 3 and 4. Further, since three adjacent sides of each die are showing, no die has two opposite faces showing, and each die is showing exactly one number out of each pair (1, 6), (2, 5), and (3, 4).
To keep track of our dicoveries, I'll make a grid, with the faces of the cube against the corners. Let U be the top, D the bottom, F for front, B for the back, and if you can't figure out L and R you shouldn't read any further. I'll name the dice after the letter of their corner, to make the following grid:
A B C D E F G H
U _ _ X X _ _ X X ....
D X X _ _ X X _ _ ....
L _ X X _ _ X X _ ....
R X _ _ X X _ _ X ....
F _ _ _ _ X X X X ....
B X X X X _ _ _ _ ....
. . . . . . . .
. . . . . . . .
. . . . . . . .
Each die is only represented on certain faces, obviously, and the '_'s in the grid will be filled in with the numbers in those positions on the cube (CD is the number on the bottom face of die C, etc). The '.'s in the grid represent the numbers on the face in that row, or the die in that column, respectively, without respect for order. That's just semantics, and probably messier than it need be, but I'm trying to represent in ASCII how I solved this on paper.
Now, to the problem. In clue 2, we are told that two of the faces contain the digits to two different four-digit squares. Luckily, there are only two four-digit squares comprised of the digits 1-6 that do not repeat any digits: 4356 (66˛) and 6241 (79˛). From clue 5, the 3456 must appear on the front, which means 1246 are on the back.
From clue 6, corner be must show 135, and the only group of distinct digits 1-6 that multiply to 180 is 2356, which are on the top. There is only one 5 showing in the top half of the cube (8), so the 5 on die B must be on top. Also, since the other three dice in the top half (A, E, F) cannot have a 5 showing, they must show a 2. There is no 1 on the front of the cube, so the 1 on die B must be on the right, and the 3 is on the front.
We also know that the numbers on the top and bottom face of the cube both add to 16.
Incorporating what we're told in clue 4, here's what we have so far:
A B C D E F G H
U _ 5 X X _ _ X X 2356
D X X _ _ X X _ _ .... (add to 16)
L _ X X _ _ X X _ ....
R X 1 _ X X _ _ X 1...
F _ 3 _ _ X X X X 3456
B X X X X _ _ _ _ 1246
. 1 . . . 6 . .
2 5 . . 2 2 . .
. 3 . . . 3 . .
Continuing on, in clue 7, the only possible numbers showing on die G are 124, and die E shows 246. Since dice A, E, F, and G all show 2s and there are 4 of each number (1), the other four dice (B, C, D, H) must show 5s.
In clue 9, there are only a few sets of numbers 1-6 that include a 5 for which one number is the sum of the other two: 1+4=5, 1+5=6, and 2+3=5. However, 1 and 6 cannot show on the same die, nor can 2 and 5, so die C shows 145.
A B C D E F G H
U _ 5 X X _ _ X X 2356
D X X _ _ X X _ _ .... (add to 16)
L _ X X _ _ X X _ ....
R X 1 _ X X _ _ X 1...
F _ 3 _ _ X X X X 3456
B X X X X _ _ _ _ 1246
. 1 1 . 6 6 1 .
2 5 5 5 2 2 2 5
. 3 4 . 4 3 4 .
The highest number on the back face of the cube is 6, and the lowest is 1. The face of E on that side must be the highest or the lowest, and since die E does not have a 1, that must be the 6. Now, there is no E on the top face, so the 2 from die E is on top and the 4 is on the left. The 4 on the left cannot be the highest (only 5 and 6 are higher) on the side; it must be the highest, and the left side shows the numbers 1234. The 2 on that left side cannot come from die D or H; it must be from A.
A B C D E F G H
U _ 5 X X 2 _ X X 2356
D X X _ _ X X _ _ .... (add to 16)
L 2 X X _ 4 X X _ 1234
R X 1 _ X X _ _ X 1...
F _ 3 _ _ X X X X 3456
B X X X X 6 _ _ _ 1246
. 1 1 . 6 6 1 .
2 5 5 5 2 2 2 5
. 3 4 . 4 3 4 .
The 5 on die H does not belong on either the left (1234) or the back (1246) of the cube; it must go on the bottom. Thus, the 5 from D cannot be on the bottom, or on the left (1234); it must be on the front.
A B C D E F G H
U _ 5 X X 2 _ X X 2356
D X X _ _ X X _ 5 5... (add to 16)
L 2 X X _ 4 X X _ 1234
R X 1 _ X X _ _ X 1...
F _ 3 _ 5 X X X X 3456
B X X X X 6 _ _ _ 1246
. 1 1 . 6 6 1 .
2 5 5 5 2 2 2 5
. 3 4 . 4 3 4 .
The 6 on die F is not on the right (clue 8) or the back (E6 is there); it is on the top. Thus, the 3 on the top comes from die A. The 3 on die F is not on the back, either; it's on the left and F shows a 2 on the back side of the cube. The remaining face of A is on the front, and it's either a 1 or a 6; since there is no 1 on the front, it's a 6. The 4 from the front must, then, come from die C.
A B C D E F G H
U 3 5 X X 2 6 X X 2356
D X X _ _ X X _ 5 5... (add to 16)
L 2 X X _ 4 X X _ 1234
R X 1 _ X X 3 _ X 13..
F 6 3 4 5 X X X X 3456
B X X X X 6 2 _ _ 1246
6 1 1 . 6 6 1 .
2 5 5 5 2 2 2 5
3 3 4 . 4 3 4 .
Also note that each digit shows up on four of the six sides. Since two sides (B 1246 and U 2356) do not have a 1, the other four (D, L, R, B) must. That puts a 1, a 5, and two other numbers on the bottom that must add to 16; and the only pair of distinct numbers that can add to 10 is 4 and 6 -- so 1456 are on the bottom. That 6 is not on die C or G, nor from H (which shows a 5 on the bottom); it's on die D. Die H must have a 1 showing. The last two numbers on the right side must be 2 and 5.
A B C D E F G H
U 3 5 X X 2 6 X X 2356
D X X _ 6 X X _ 5 1456
L 2 X X _ 4 X X _ 1234
R X 1 _ X X 3 _ X 1235
F 6 3 4 5 X X X X 3456
B X X X X 6 2 _ _ 1246
6 1 1 6 6 6 1 1
2 5 5 5 2 2 2 5
3 3 4 . 4 3 4 .
The last face of die D must be a 3 or a 4 and show on the left side; since the left already shows a 4 (die E), it must be a 3. The 1 on the left, then, comes from die H. The last face of die H is a 4 (dice A, B, D, and F have the four threes), which is on the back of the cube.
A B C D E F G H
U 3 5 X X 2 6 X X 2356
D X X _ 6 X X 4 5 1456
L 2 X X 3 4 X X 1 1234
R X 1 _ X X 3 2 X 1235
F 6 3 4 5 X X X X 3456
B X X X X 6 2 1 4 1246
6 1 1 6 6 6 1 1
2 5 5 5 2 2 2 5
3 3 4 3 4 3 4 4
Now, just to clean up: the 1 on the back must come from die G. The face of G on the right can't be the 4; it's the 2, and G shows the 4 on the bottom. The 1 on the bottom and the 5 on the right side, then, both come from die C.
A B C D E F G H
U 3 5 X X 2 6 X X 2356
D X X 1 6 X X 4 5 1456
L 2 X X 3 4 X X 1 1234
R X 1 5 X X 3 2 X 1235
F 6 3 4 5 X X X X 3456
B X X X X 6 2 1 4 1246
6 1 1 6 6 6 1 1
2 5 5 5 2 2 2 5
3 3 4 3 4 3 4 4
And, we're done! I'm not sure how best to display the results, except maybe to show what the cube would look like unfolded. The top is on top, the bottom on the bottom, the front in the center, the back to the far right, and again, if you can't figure out left and right from there, I feel bad for you:
2 6
3 5
4 2 6 3 1 3 2 6
1 3 5 4 5 2 1 4
6 1
5 4
|
|
Posted by DJ
on 2004-12-22 18:02:03 |