Three circles (A, B, and C) are tangentially connected:

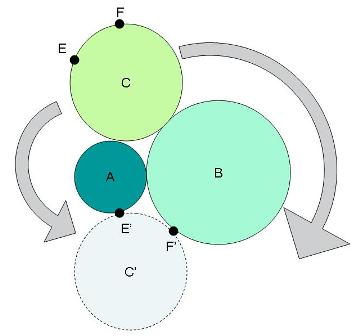
Properties:
1) Circle B's radius is twice the radius of Circle A.
2) Circle C is of the exact size that if it rolls around A, or it rolls around B, it will touch A and B on the opposite side at identical points E and F.

What is the radius of Circle C if:
1) C > B
2) B > C > A
3) A > C (C is a maximum)

If circle A's radius is given as 1, then circle B's radius is 2 and circle C's radius is c, where c is the current unknown.

If the center points, a, b, and c, of each respective circle, A, B and C, were connected by line segments a triangle would be formed. Segment ac would have a length of (1+c); segment bc would have a length of (2+c); and segment ab would have a length of 3, (1+2).

Using the law of cosines and in terms of the radial length c, we can find angle cab equal to
cos^-1((3-c)/(3+3c)),
and angle cba equal to
cos^-1((6+c)/(6+3c)).

The arc segment of each circle, A and B, untouched by rolling circle C, would then be equal to twice the corresponding angle multiplied by it corresponding radius. Subtracting each arc segment length from the circumference of each respective circle, A and B, would give the distance C is rolled around both circles A and B. This distance, D, is then equal to
4(pi - cos^-1((6+c)/(6+3c))) + 2(pi - cos^-1((3-c)/(3+3c)))

To find c, then, we seek a solution where the number R revolutions of circle C around both circles A and B make one complete circuit and where c is a multiple of R and/or R is a multiple of c. That is, where (2*pi*c)/D or D/(2*pi*c) is an integer. And where, for the corresponding goal where C > B;
B > C > A; and A > C (C is a maximum).

Edited on December 27, 2007, 9:20 pm

Posted by Dej Mar on 2007-12-27 19:26:12