An octagonal number is a number that is represented by the set of figures given below.
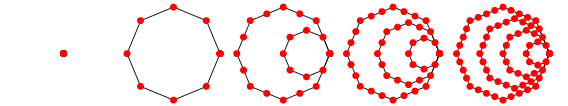
A certain octagonal number is the sum of the squares of three terms in an arithmetic sequence with a common difference of 704. What is that octagonal number?
|
|
Submitted by K Sengupta
|
|
Rating: 5.0000 (1 votes)
|
|
|
Solution:
|
(Hide)
|
The required number is 25,119,920.
EXPLANATION:
Let us suppose that the three consecutive terms of the Arithmetic Series under reference are (y-704), y and (y+704).
Accordingly, we obtain:
x(3x-2) = (y-704) + y^2 + (y+704)^2
or, (3x-1)^2 = (3y)^2 + 2973697
Accordingly, (3x+3y-1, 3x-3y-1) = (17189,173).
or, (3x + 3y - 1)(3x - 3y -1)=2973697*1 = 17189 * 173
Hence; (3x + 3y -1, 3x - 3y -1)=(2973697, 1),(17189, 173)
In conformity with provisions of the problem under reference, both x and y are positive integers. Now, we observe that 3x- 3y -1 = 1 gives
x-y = 2/3, which is a contradiction.
Hence, (3x + 3y -1, 3x - 3y -1)=(17189, 173)
Solving the above simultaneous equation, we obtain (x,y)=(2894,2836), so that the three consecutive terms of the Arithmetic Series are 2132,2836 and 3540.
Consequently:
2894th Octagonal Number
= 2894(3*2894 - 2)
= 25119920
=2132^2 +2836^2 +3540^2
Hence, the required number is 25,119,920.
|



