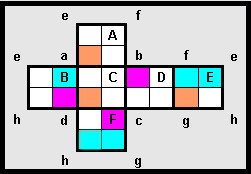
I applied one of the digits 1 through 9 to each cell of the provided net of a cube.
My object was to create a unique 4 digit square number on each face. At the same time I required each vertex to be a 3 digit square. I failed in that objective!
I have 6 unique 4 digit squares but I have duplicated just one of my vertices.
To emulate my "feat":
- a [Magenta]
Magenta cell is both the first digit of a 3 and 4 digit square
- an [Orange]
Orange cell signifies the first digit of only a 4 digit square, while
- a [Cyan]
Cyan cell signifies the first cell only of a 3 digit square.
The digits must be applied to each face by rotation, the direction is defined by need.
"A" through "F" represent the 6 faces of the cube while "a" through "h" represent the vertices of the cube when fully assembled.
 Note:
Note: Within the range allowed several squares utilise the same digits, and this is allowed by virtue of the commencement cell.
But then, there is still the challenge for 6 unique faces and 8 unique vertices.
(In reply to
re: Computer solutions by Charlie)
After posting my solutions, I looked at the proposed official solution in the solution queue. This made me recognize that not all my anomalous counts (more than 8 vertex squares counted) were as innocuous as the one presented in my first post.
Specifically, to take an example:
98
68
65744921
16744896
61
92
6889 6561 7744 4489 9216 1296
576 484 144 676 289 961
144 1 169 1 196 1 289 2 484 1 576 1 676 1 961 1
98
68
65744921
16744896
61
92
6889 6561 7744 4489 9216 1296
576 484 144 676 289 961
144 1 169 1 196 1 289 2 484 1 576 1 676 1 961 1
First of all, while this purports to be two solutions, it's really only one: the faces A through F are the same, as are the other numbers.
Second, 289 is indeed unavoidably repeated, and it is the 169 and 196 that account for the total set of squares exceeding 8, and they are at the same vertex.
This does not affect the non-anomalous solutions, and only some of the anomalous ones. (anomalous means total vertex squares exceed 8).
|
|
Posted by Charlie
on 2008-05-02 17:36:42 |




